Grade - 4 Math
Average
In our daily conversations, we often use the word ‘average’. Without really understanding the meaning of it. The meaning is really simple.
An average is a single number that gives an indication of most numbers or quantities in a group.
Consider the following situation.
Following are the marks obtained by a group of students of a particular class in a mathematics exam.
The sum of the marks obtained by the five students is 400. If we divide the sum of the marks by 5, we get the average marks obtained by the five students.
Average marks obtained = 400 / 5 = 80
This does not mean that all the students scored exactly 80 marks. Some of the students have scored more than 80 marks while some of them scored less than 80 marks, but by and large, most students scored around 80. The average marks tell us how many marks each student would have scored if all students scored the same marks.
The average lies between the greatest and the least quantity.
The average of a given number of quantities need not be a value in the given set of quantities.
Average cannot be calculated from two or more different types of quantities.
An average is a single number that gives an indication of most numbers or quantities in a group.
The average of two or more quantities is the sum of all the quantities divided by the number of quantities.
Average = Sum of the quantities / Number of quantities
Q. The weight of 6 books is 924 grams. Find the average weight of these books.
Solution:
The weight of 6 books is 924 gram
The average weight of books = ( 924 / 6 ) gram
= 154 gram Ans.
Q. Find the average of x + y, 2x + 3y, 2x – 2y, and 3x – 2y.
( x + y ) + (2x + 3y) + ( 2x – 2y) + ( 3x – 2y) = 8x
The average = 8x / 4 = 2x.
If the speed of an object does not change throughout its journey, the object is said to be traveling at a constant speed.
However, in real-life situations, it is unlikely for an object to travel at the same speed throughout its journey. Consider the speed of the ferry in worked.
The average speed of an object is defined as the total distance traveled by the object per unit of time.
International Place- Value System:
A different form of place-value chart known as the International Place-value Chart is used in most other countries of the world.
Million Thousands Ones
Hundred - Ten - Million Hundred - Ten - Thousands - Hundreds - Tens - Ones
millions - millions - thousands -thousands -
84349132
72456234
* The first three places from the right are the 'ones' period.
* The next three places are in the 'thousands' period.
* The next three places are in the 'millions' period.
Rounding Number:
* When we round a number to the nearest ten, we round it to the multiple of ten nearest to it.
* A number which is midway is always rounded up.
* When we round a number to the nearest hundred, we round it to the multiple of hundred nearest to the number.
* A number which is midway is always rounded up.
Exercise:
a. Round the following numbers to the nearest ten.
1. 178
2. 56
3. 71
4. 49
5. 25
b. What numbers can be rounded to the nearest 10 to:
1. 140
2. 200
3. 1000
c. Round the following numbers to the nearest hundred.
1. 1289
2. 535
3. 1229
d. Round the following numbers to the nearest thousands.
1. 13,546
2. 24,408
3. 36,705
Roman Numerals:
There is no zero in the Roman system. An important difference between the decimal system and the Roman system is that the Roman system does not use place value.
Rules:
1. Numerals I, X, C and M can be repeated to represent a number. Repetition means addition. I, X and C can not be repeated more than 3 times. Symbols V, L, D are not repeated.
Thus, III = 3
XXX = 30
CCC = 300
2. A smaller number written to the right of the numeral of greater value is always added to the greater numeral.
VI = 5 + 1 = 6
XV = 10 + 5 = 15
LX = 50 + 10 = 60
CX = 100 + 10 = 110
3. A smaller number written to the left of a number of greater value is always subtracted from the greater numeral.
IV = 5 - 1 = 4
XL = 50 - 10 = 40
XC = 100 - 10 = 90
4. When a smaller number is placed between two numerals of greater value, it is always subtracted from the greater numeral immediately following it.
XIV = 10 + (5 - 1 )
= 14
XXIX = 10 + 10 + ( 10 -1 )
= 29
CXXIV = 100 + 10 + 10 + (5 - 1 )
= 124
Ex. Write the following in Roman numerals.
a) 48
b) 77
c) 98
Estimating Sums:
Ex. 1. There are 92 coins in box A and 47 in box B. Estimate the total number of coins in both the boxes.
Let us round off the numbers to the nearest ten and add
92 ------------.> 90
47----------- > 50
---------------
140
There are about 140 coins in both the boxes together.
92 + 47 = 139
Actual number of coins is 139.
the estimate 140 differs from the actual 139 by only 1.
Ex. 2. The number of trees in two farms are 168 and 314. Estimate the total number of trees in both the farms taken together.
Word Problem on Addition:
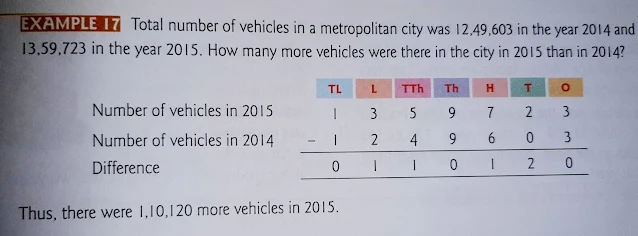
Ex. 1. A large public library has 233,456 books in English and 7,25, 608 books in other languages. How many books does the library have?
Solution:
Number of books in English = 23456
Number of books in other languages = 725608
--------------------------------------------------------------------
Total number of books = 749064
Ans: 7,49,064 books.
Ex. 2. There are 2,38,466 men, 2,41,588 women and 1,08,350 children in a town. What is the population of the town?
Solution:
Number of men in the town = 238466
Number of women in the town = 241588
Number of children in the town = 108350
----------------------------------------------------
Population of the town = 588304
The largest 6 - digit number is - 999999
The smallest 6- digit nunmber is - 1000000
Subtraction:
The number which is subtracted is called the subtrahend.
The number from which the subtrahend is subtracted is called the minuend.
The result we get after the subtraction is called the difference.
Word Problems:
Ex.1. The population of Ramgarh is 1,38,460 and the population of Feni is 2,32,560. Which town has greater population? How much more ?
Population of Feni = 232560
Population of Ramgarh = 138460
-------------------------------------------------------------
The population of Feni is more by = 94100
Ans: 94100
Ex.2. The sum of two numbers is 5,65,400. If one of them is 2,38,505, find the other number.
(5,65,400 - 2,38,505) = 326895
Ans: The other number is 3,26,895
Ex. 1. There were 5,639 people in a train. 1,059 people got down at Station A and 595 people got down at station B. How many people are left in the train?
Number who got down at station A = 1059
Number who got down at station B = 595
--------------------------------------------------------
Total = 1654
Number who were on the train = 5639
Total number that got down = 1654
--------------------------------------------------------
Number of people left = 3885
Answer: 3885.
Multiplication:
Word Problem:
Ex.1. The apples grown in a farm were packed in 302 boxes. Each box contained 256 apples. How many apples were there in all?
Solution:
1 box contains = 256 apples
302 boxes contain = (256 X 302)
= 77312 apples
Division Word Problem:
Ex. 1. The distance covered by a car in 5 hours is 200 km. What is the distance covered by the car in 3 hours?
Solution:
Distance covered by the car in 1 hour = 200 km / 5 = 40km
Distance covered by the car in 3 hours = 40km x 3 = 1220km
Factors and Multiples
Properties of multiples
1. Every number is a multiple of itself. Every number is also a multiple of 1.
2. A multiple of a number is always greater than or equal to the number itself.
3. A number has an unlimited of multiples
Odd and even numbers
A number which is a multiple of 2 is called an even number.
Thus 2, 4, 6, 8, .......... are even numbers
A number which is not a multiple of 2 is an odd number.
1, 3, 5, 7, ........... are odd numbers.
Exercise 1
A. What are the next five multiples of the following numbers?
1) 4
2) 7
3) 10
4) 2
5) 5
B. Say whether true or false.
1. Every number is a multiple of itself.
2. Every number is not a multiple of 1.
3. 20 is a multiple of 1.
4. A multiple of a number cannot be equal to the number itself.
5. A multiple of a number can be greater than the number itself.
C. Fill in the blanks.
1) 3 X 4 = 12. 12 is a multiple of __4__ and _6__.
2) 7 X 3 = 21. 21 is a multiple of 3 and 4 .
3) 4 X 5 = 20. 20 is a multiple of 4 and 5 .
4) 7 X 9 = 63 . 63 is a multiple of 7 and 9 .
5) 9 X 8 = 72. 72 is a multiple of 9 and 8 .
6) 8 X 8 = 64. 64 is a multiple of 8 .
D. 1) Write the next three multiples.
a) 6, 12, 18, 24 , 30 , 36 .
b) 5, 10, 15, 20, 25 , 30 , 35 .
c) 9, 18, 27 , 36 , 45 .
2) Fill in the missing multiples
a) 7, 14, 21 , 28, 35
b) 8 , 16, 24, 32, 40 , 48
EM Book
Ex. 8.8
6. Sabuj's house is 3/8 km to the west of the school. Mitu's house is 5/12 km to the east of her house.
a) How many km is it from Sabuj's house to Mitu's house?
b) Whose house is nearer to school? And how many km is the difference?
solution: Sabuj house is 3/8 km to the west of school
Mitu's house is 5/12 km to the east of school
So, the school is (3/8 + 5/12) km from Sabuj;s house to Mitu's house
= (9+10/24)km
= 19/24 km Ans.
b) Here, Sabuj's house is 3/8 km from school & Mitu's house is 5/12 km from school L.C.M of 8 and 12 is 24.
24 / 8 = 3
∴ 3/8 = 3.3/8.3
= 9/24
24/12 = 2
∴ 5/12 = 5.2/12.2
= 10/24
9< 10
9/24 < 10/24
3/8 < 5/12
Sabuj's house is nearer to school.
Difference of the distance = (5/12 - 3/8) km
= ( 10-9 / 24) km
= 1/24 km
7. A farmer planted brinjal in 1/2 part, cabbage in 1/4 part and flowers in 1/5 part of his garden.
a) How much part of garden did he plant?
b) How much part of the garden remained blank?
Solution:
a) The part of land in which he planted = (1/2 + 1/4 + 1/5)
= (10+5+4)/20
= 19/20
b) Part of the garden which remained blank = ( 1 - 19/20)
= 20 - 19 / 20
= 1/20 part Ans.